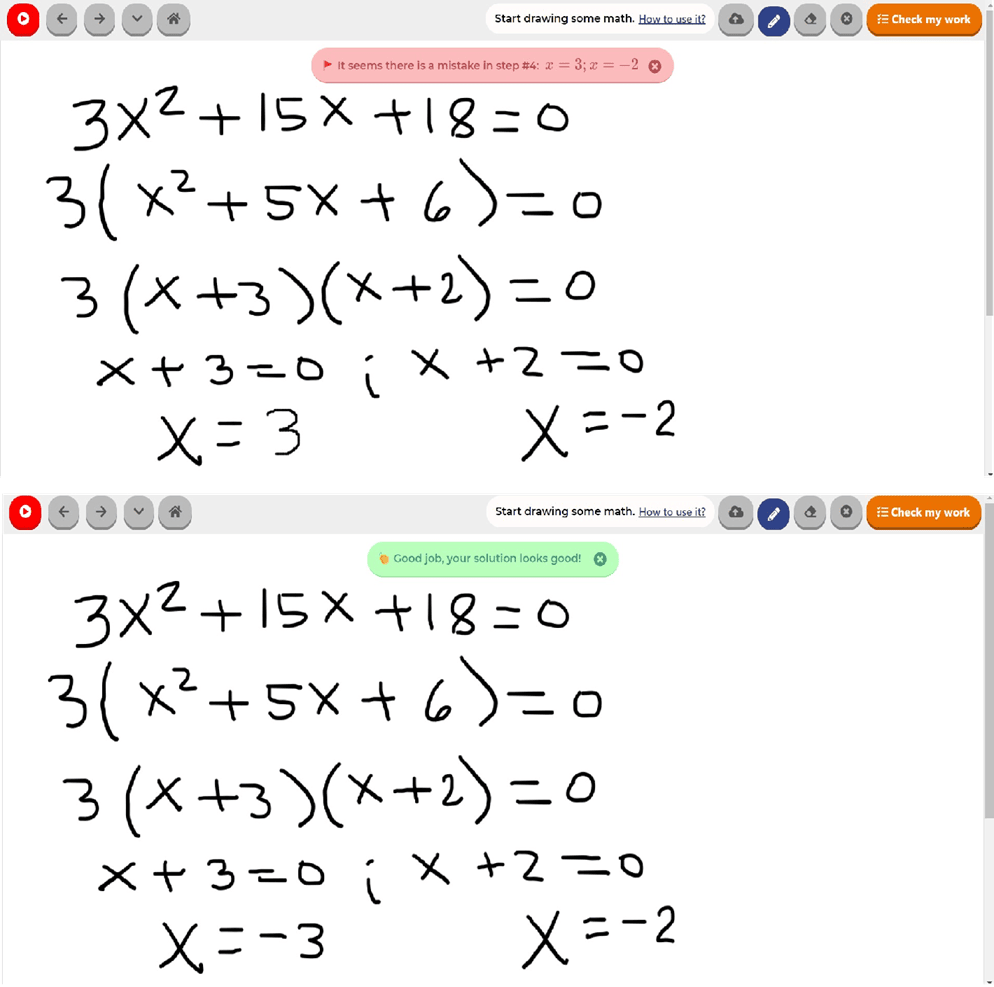
Final answer to the problem
Step-by-step Solution
Apply the formula: $a\log_{b}\left(x\right)$$=\log_{b}\left(x^a\right)$, where $a=64$, $b=10$ and $x=4$
Apply the formula: $a\log_{b}\left(x\right)$$=\log_{b}\left(x^a\right)$, where $a=64$, $b=10$ and $x=4$
Solving a math problem using different methods is important because it enhances understanding, encourages critical thinking, allows for multiple solutions, and develops problem-solving strategies. Read more
Combining or condensing logarithms consists of rewriting a mathematical expression with several logarithms into a single logarithm, by applying the properties of logarithms.
Get a preview of step-by-step solutions.
Get 3 free complete solutions daily when you signup with your student or academic email.
Earn solution credits, which you can redeem for complete step-by-step solutions.
Save your favorite problems.
Become premium to access unlimited solutions, downloads, discounts and more!