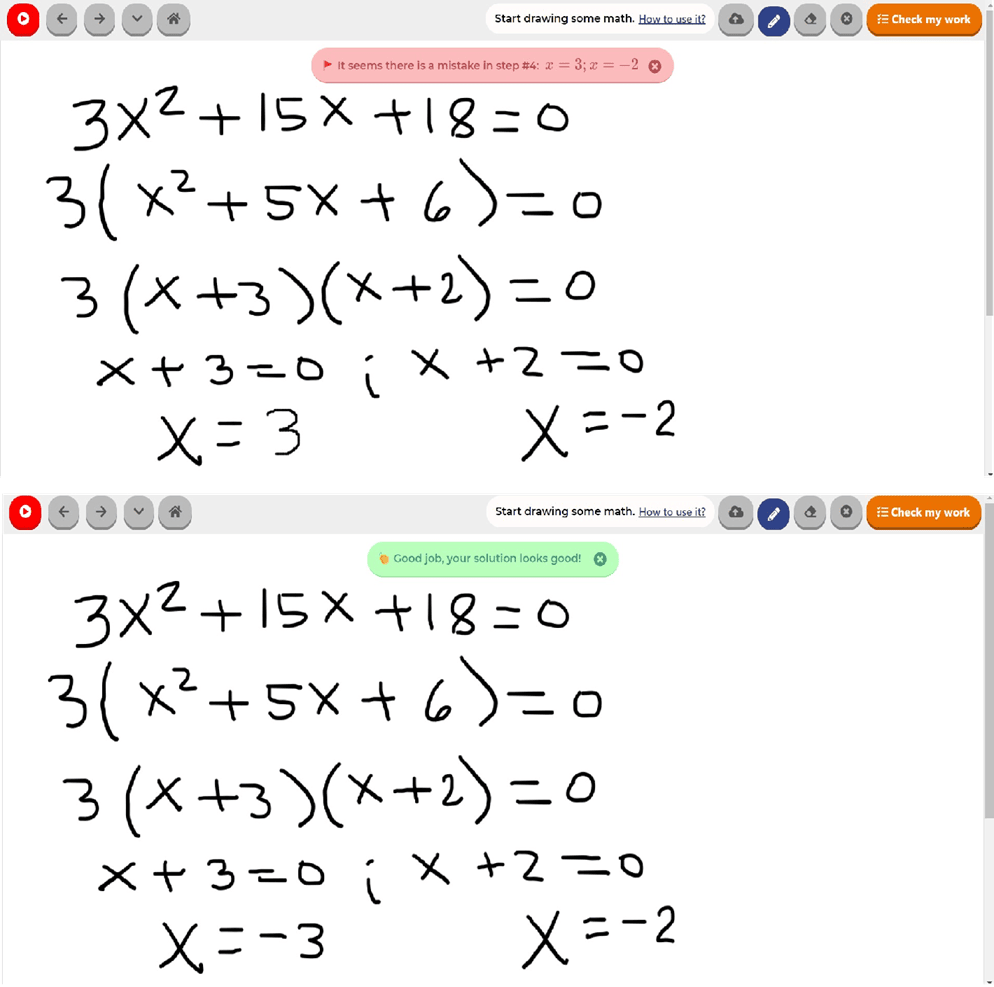
Final answer to the problem
$\frac{4x^{4}\ln\left|x\right|^2-2x^{4}\ln\left|x\right|+\frac{1}{2}x^{4}}{16\cdot \ln\left|10\right|^2}+C_0$
Got another answer? Verify it here!
Step-by-step Solution
Learn how to solve integration techniques problems step by step online. Solve the integral of logarithmic functions int(x^3log(x)^2)dx. Change the logarithm to base e applying the change of base formula for logarithms: \log_b(a)=\frac{\log_x(a)}{\log_x(b)}. Simplify the expression. Take the constant \frac{1}{\ln\left|10\right|^2} out of the integral. We can solve the integral \int\ln\left(x\right)^2x^3dx by applying integration by parts method to calculate the integral of the product of two functions, using the following formula.
Final answer to the problem
$\frac{4x^{4}\ln\left|x\right|^2-2x^{4}\ln\left|x\right|+\frac{1}{2}x^{4}}{16\cdot \ln\left|10\right|^2}+C_0$
