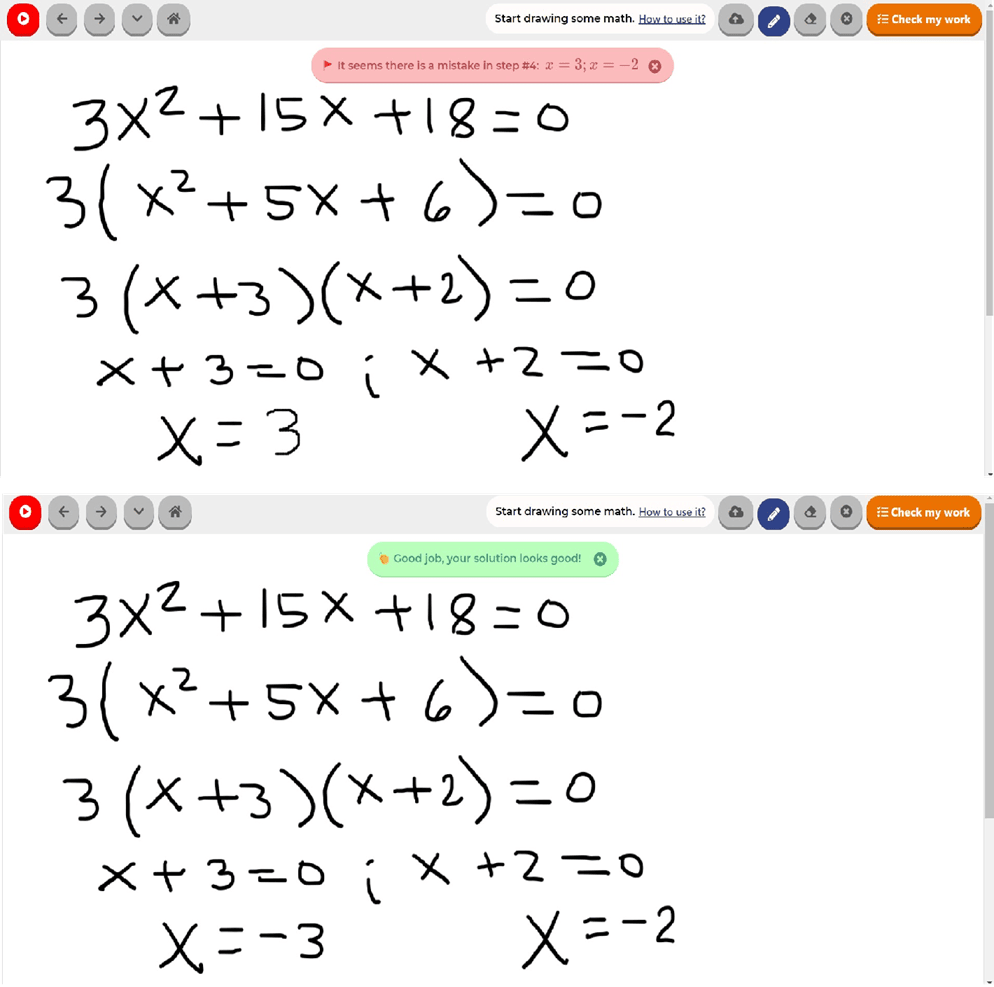
Final answer to the problem
$\frac{x\left(x-2\right)}{3\left(x-3\right)\left(x-4\right)}$
Got another answer? Verify it here!
Step-by-step Solution
Learn how to solve equivalent expressions problems step by step online. Simplify the expression (x^2+2x)/(3x^2-18x+24)(x^2-4x+4)/(x^2-x+-6). Multiplying fractions \frac{x^2+2x}{3x^2-18x+24} \times \frac{x^2-4x+4}{x^2-x-6}. Factor the trinomial \left(x^2-x-6\right) finding two numbers that multiply to form -6 and added form -1. Rewrite the polynomial as the product of two binomials consisting of the sum of the variable and the found values. The trinomial \left(x^2-4x+4\right) is a perfect square trinomial, because it's discriminant is equal to zero.
Final answer to the problem
$\frac{x\left(x-2\right)}{3\left(x-3\right)\left(x-4\right)}$
