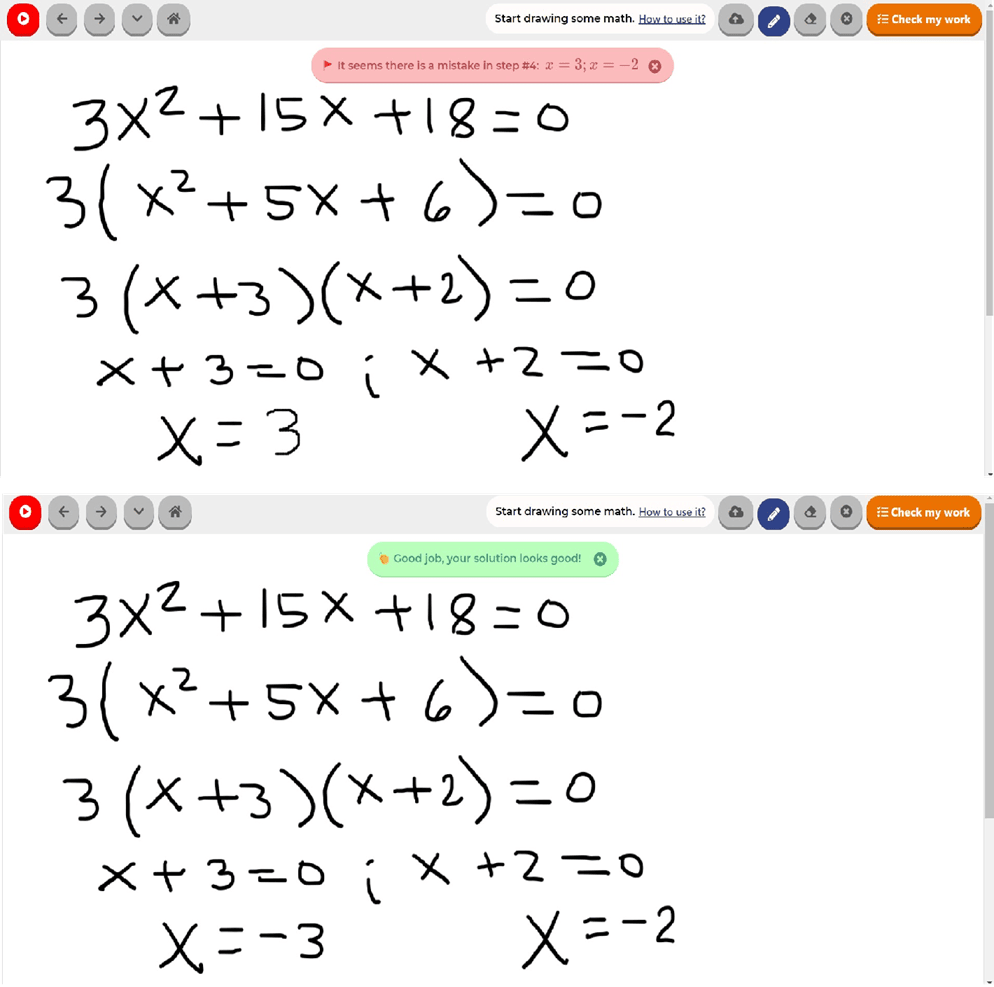
Final answer to the problem
Step-by-step Solution
To derive the function $\left(x^2+3\right)^{\left(5x-1\right)}$, use the method of logarithmic differentiation. First, assign the function to $y$, then take the natural logarithm of both sides of the equation
Apply natural logarithm to both sides of the equality
Using the power rule of logarithms: $\log_a(x^n)=n\cdot\log_a(x)$
Derive both sides of the equality with respect to $x$
Apply the product rule for differentiation: $(f\cdot g)'=f'\cdot g+f\cdot g'$, where $f=5x-1$ and $g=\ln\left(x^2+3\right)$
The derivative of the natural logarithm of a function is equal to the derivative of the function divided by that function. If $f(x)=ln\:a$ (where $a$ is a function of $x$), then $\displaystyle f'(x)=\frac{a'}{a}$
The derivative of the natural logarithm of a function is equal to the derivative of the function divided by that function. If $f(x)=ln\:a$ (where $a$ is a function of $x$), then $\displaystyle f'(x)=\frac{a'}{a}$
The derivative of the linear function is equal to $1$
The derivative of the constant function ($-1$) is equal to zero
The derivative of a sum of two or more functions is the sum of the derivatives of each function
The derivative of the constant function ($3$) is equal to zero
The derivative of a sum of two or more functions is the sum of the derivatives of each function
The derivative of a function multiplied by a constant is equal to the constant times the derivative of the function
The derivative of the linear function is equal to $1$
The derivative of the linear function times a constant, is equal to the constant
The derivative of the linear function is equal to $1$
The power rule for differentiation states that if $n$ is a real number and $f(x) = x^n$, then $f'(x) = nx^{n-1}$
Subtract the values $2$ and $-1$
The power rule for differentiation states that if $n$ is a real number and $f(x) = x^n$, then $f'(x) = nx^{n-1}$
Multiply the fraction by the term
Any expression multiplied by $1$ is equal to itself
Multiply the fraction by the term
Multiply both sides of the equation by $y$
Substitute $y$ for the original function: $\left(x^2+3\right)^{\left(5x-1\right)}$
The derivative of the function results in
Combine all terms into a single fraction with $x^2+3$ as common denominator
Solve the product $5\left(x^2+3\right)\ln\left(x^2+3\right)$
Multiply $5$ times $3$
Solve the product $2\left(5x-1\right)x$
Solve the product $5\left(x^2+3\right)\ln\left(x^2+3\right)$
Multiply $5$ times $3$
Multiply $2$ times $5$
Multiply $2$ times $-1$
When multiplying two powers that have the same base ($x$), you can add the exponents
Solve the product $2\left(5x-1\right)x$
When multiplying two powers that have the same base ($x$), you can add the exponents
Multiplying the fraction by $\left(x^2+3\right)^{\left(5x-1\right)}$
Simplify the fraction $\frac{\left(5x^2\ln\left(x^2+3\right)+15\ln\left(x^2+3\right)+10x^2-2x\right)\left(x^2+3\right)^{\left(5x-1\right)}}{x^2+3}$ by $x^2+3$
Simplify the derivative