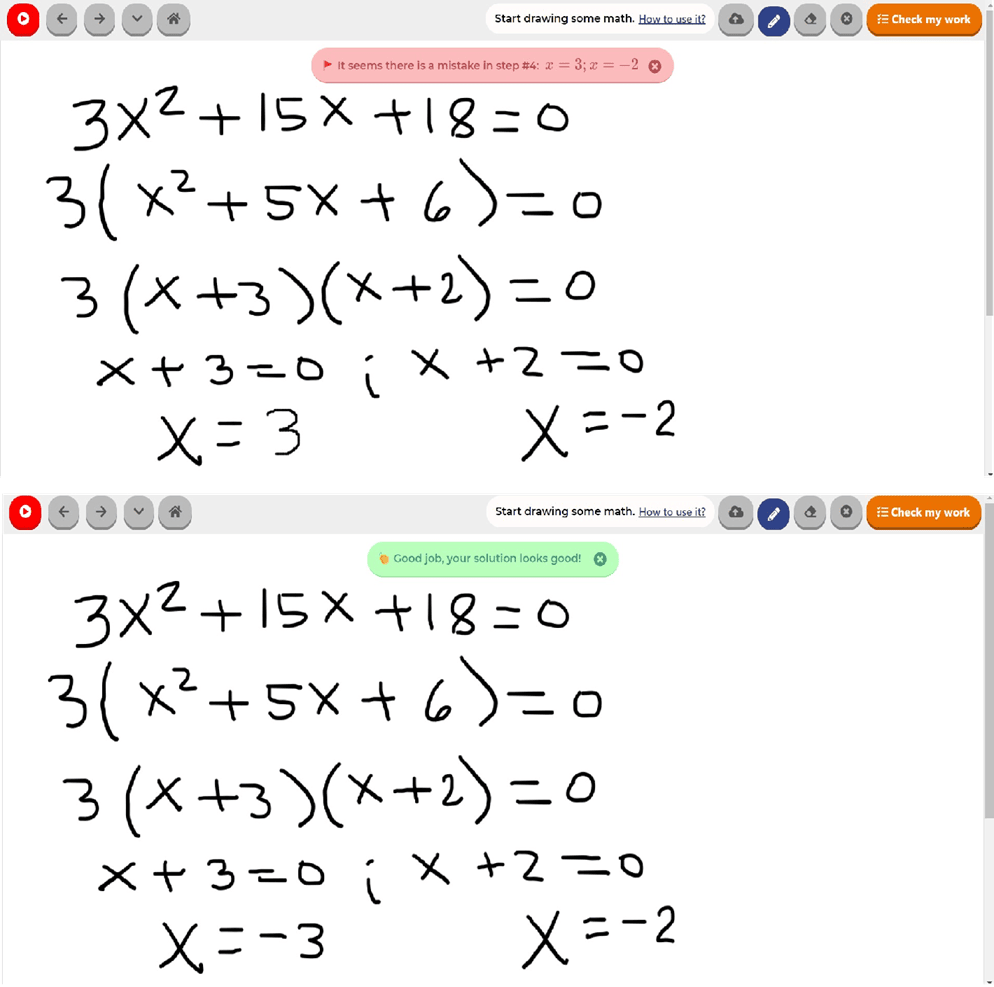
Final answer to the problem
$y=\frac{\sqrt{\ln\left(c\right)}}{\sqrt{x}},\:y=\frac{-\sqrt{\ln\left(c\right)}}{\sqrt{x}}$
Got another answer? Verify it here!
Step-by-step Solution
How should I solve this problem?
- Choose an option
- Solve for x
- Solve for y
- Solve for c
- Find the derivative
- Solve by implicit differentiation
- Solve for y'
- Find dy/dx
- Derivative
- Find the derivative using the definition
- Load more...
Can't find a method? Tell us so we can add it.
1
We can take out the unknown from the exponent by applying natural logarithm to both sides of the equation
Learn how to solve exponential equations problems step by step online.
$\ln\left(e^{xy^2}\right)=\ln\left(c\right)$
Learn how to solve exponential equations problems step by step online. Solve the exponential equation e^(xy^2)=c. We can take out the unknown from the exponent by applying natural logarithm to both sides of the equation. Apply the formula: \ln\left(e^x\right)=x, where x=xy^2. Divide both sides of the equation by x. Removing the variable's exponent.
Final answer to the problem
$y=\frac{\sqrt{\ln\left(c\right)}}{\sqrt{x}},\:y=\frac{-\sqrt{\ln\left(c\right)}}{\sqrt{x}}$
