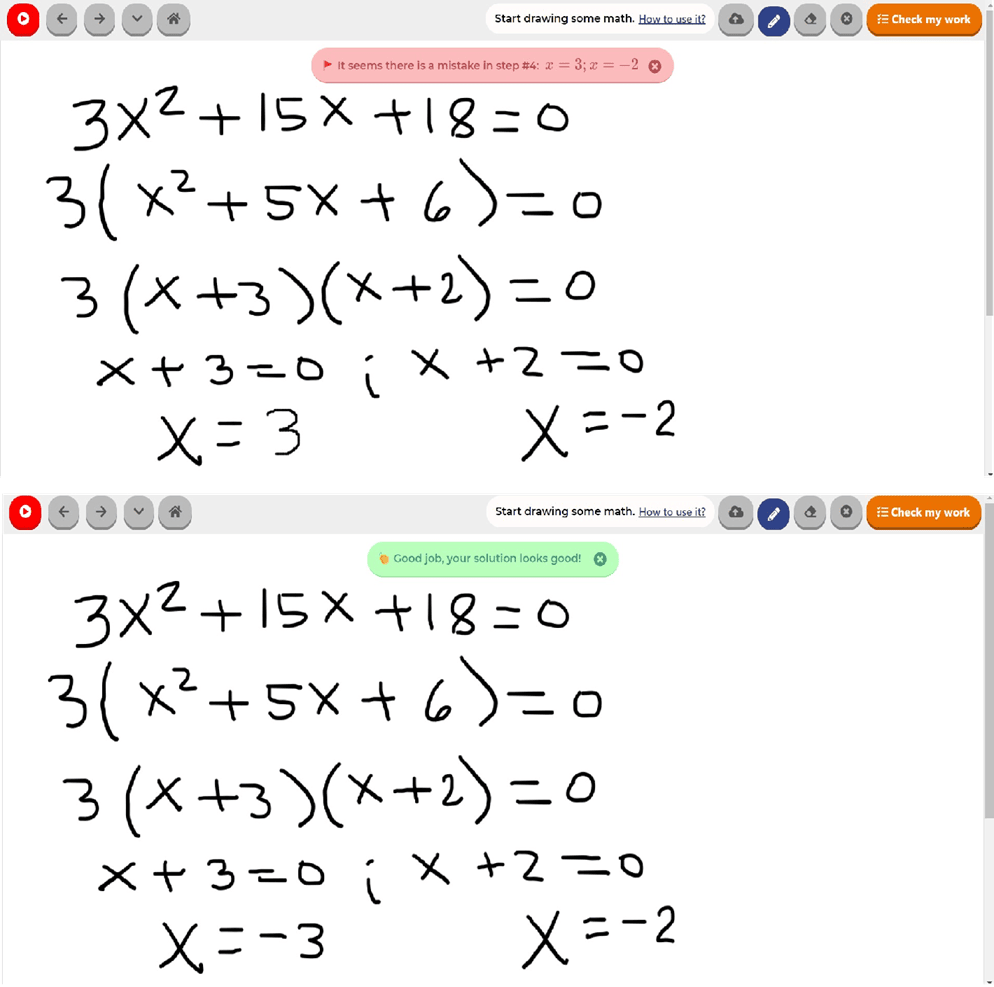
Final answer to the problem
$y=\frac{\ln\left(\frac{1}{2}\right)}{x}$
Got another answer? Verify it here!
Step-by-step Solution
How should I solve this problem?
- Choose an option
- Solve for x
- Solve for y
- Find the derivative
- Solve by implicit differentiation
- Solve for y'
- Find dy/dx
- Derivative
- Find the derivative using the definition
- Solve by quadratic formula (general formula)
- Load more...
Can't find a method? Tell us so we can add it.
1
Divide both sides of the equation by $2$
Learn how to solve problems step by step online.
$e^{xy}=\frac{1}{2}$
Learn how to solve problems step by step online. Solve the exponential equation 2e^(xy)=1. Divide both sides of the equation by 2. We can take out the unknown from the exponent by applying natural logarithm to both sides of the equation. Apply the formula: \ln\left(e^x\right)=x, where x=xy. Divide both sides of the equation by x.
Final answer to the problem
$y=\frac{\ln\left(\frac{1}{2}\right)}{x}$
